Jcrabapple @jcrabapple

1 post1 participant0 posts today

**Phracker** @Phracker2Art@mstdn.social · 1d *

1d *

Phracker @Phracker2Art@mstdn.social

Synthesis

Multiple concentric rings of rainbow-colored shapes in front of a black background with DOS characters of different colors

#Computer #ComputerArt #Digital

Continued thread

**Kake** @kake@sunny.garden · Apr 2

Apr 2

Kake @kake@sunny.garden

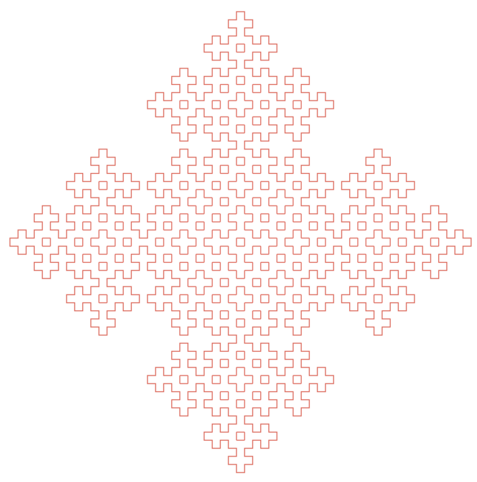
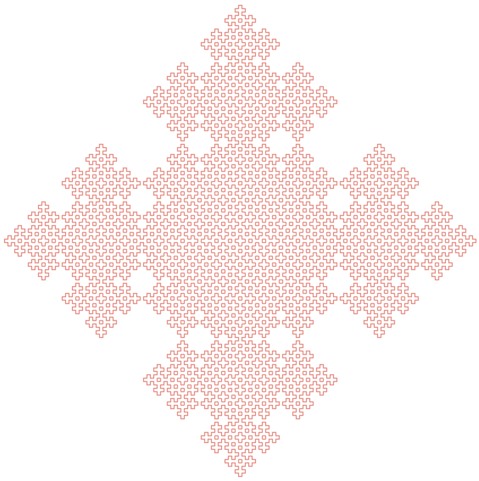
Added Math::Polygon to my Perl and I can now generate the Fibonacci snowflakes including their sashiko innards. Here are the order 5 and 6 snowflakes.

$The order 5 Fibonacci snowflake — a fractal outline made from small line segments at right angles to each other. The overall shape is rather like a square standing on one corner with bites of various sizes taken out of it. Inside the outline are snowflakes of lower orders, including the order 1 snowflake (a square) and the order 2 snowflake (a plus sign formed from 5 squares).$

The order 6 snowflake — like its order 5 counterpart, but much more complex. This is also filled with a bunch of lower-order snowflakes, but despite the complexity it can all be stitched with horizontal and vertical lines of running stitch (though practically speaking you would probably want to carry the thread across the back in some cases to avoid having too many “ends”).

#MathsArt #Sashiko #Perl

**Kake** @kake@sunny.garden · Apr 1

Apr 1

Kake @kake@sunny.garden

A while back, @gwenbeads posted about the book “A Stitch in Line: Mathematics and One-Stitch Sashiko” by Katherine Seaton, and it was so relevant to my interests I had to get hold of it. I’ve been working through it ever since, stitching the samplers and playing with the maths.

I’m currently paused at the end of chapter 8 as I wait for new thread (Retors d’Alsace!!!) to arrive for the next sampler. Here’s a photo of all my samplers so far, done on my hand-dyed muslin scraps; my plan is to patchwork them all together into a hanging once I’ve finished.

Gwen’s review of the book is in a paywalled journal, but she’s put a link to free copies (of the review, not the book) here: https://mathstodon.xyz/@gwenbeads/114094206288896863

$Seventeen small-to-medium square and rectangular pieces of cotton fabric dyed in shades of pink, purple, and blue, laid out on a cutting mat. Hitomezashi designs have been stitched on them in coordinating colours: crosses, squares, and fractal-looking designs, some regular and some random.$

#MathArt #MathsArt #Embroidery

**foldworks** @foldworks@mathstodon.xyz · Mar 25

Mar 25

foldworks @foldworks@mathstodon.xyz

Street decoration featuring eight-pointed stars, Buôn Ma Thuột, Vietnam
#Tiling #TilingTuesday #Pattern #Geometry #MathArt #MathsArt

A photograph of a pattern made of squares, triangles, parallelograms and concave pentagons. Eight-pointed stars can be perceived.

**AdeptVeritatis** @AdeptVeritatis@social.tchncs.de · Mar 15

Mar 15

AdeptVeritatis @AdeptVeritatis@social.tchncs.de

#KaleidoSaturday

4th part

See you next week!

6-fold symmetry mandala mixed into a 4-fold symmetry kaleidoscope

#Art #GenerativeArt #kaleidoscope

Continued thread

**AdeptVeritatis** @AdeptVeritatis@social.tchncs.de · Mar 15 *

Mar 15 *

AdeptVeritatis @AdeptVeritatis@social.tchncs.de

#KaleidoSaturday

3rd part with a smaller section

#Art #GenerativeArt #kaleidoscope

Continued thread

**AdeptVeritatis** @AdeptVeritatis@social.tchncs.de · Mar 15

Mar 15

AdeptVeritatis @AdeptVeritatis@social.tchncs.de

#KaleidoSaturday

2nd part

#Art #GenerativeArt #kaleidoscope

**AdeptVeritatis** @AdeptVeritatis@social.tchncs.de · Mar 15

Mar 15

AdeptVeritatis @AdeptVeritatis@social.tchncs.de

#KaleidoSaturday

Too many nice pictures today. It is a 6-fold symmetry mandala mixed into a 4-fold kaleidoscope.

Enjoy your weekend and the approaching spring.

#Art #GenerativeArt #kaleidoscope

**Her Maths Story** @hermathsstory@mathstodon.xyz · Mar 14

Mar 14

Her Maths Story @hermathsstory@mathstodon.xyz

This beautiful crocheted Richmond minimal surface - crafted by Hanne Kekkonen (who we had the honour to feature already: https://hermathsstory.eu/hanne-kekkonen/) and featured in [] - is a perfect example of how mathematics and arts intertwine.

And what better occasion to celebrate this than Pi Day - the International Day of Mathematics? This year’s theme “Mathematics, Art, and Creativity” highlights how maths shapes the world around us in unexpected and artistic ways.

Want to explore more? Here are five fascinating reads on π, where we rate the maths proficiency level from (suitable for everyone) to (for maths geeks):

Pi, explained! https://www.snexplores.org/article/lets-learn-about-pi
What is pi, and why does it pop up everywhere? A beginner-friendly dive into the world’s most famous irrational number.

When maths meets arts https://www.snexplores.org/article/math-is-muse-for-these-artists
From sculptures to paintings - how artists use mathematical ideas to create stunning works

How pi took over the world https://www.popularmechanics.com/science/math/g26630324/what-is-pi/
A whirlwind history of pi, from ancient times to cutting-edge research.

7 maths facts that will blow your mind https://www.newscientist.com/article/2418890-these-7-mathematical-facts-will-blow-your-mind/
Pi is just the beginning - get ready for some mind-bending maths!

Pi and... string theory?! https://www.scientificamerican.com/article/string-theorists-accidentally-find-a-new-formula-for-pi/
Physicists accidentally discovered a new formula for pi - while working on the universe’s deepest mysteries.

Maths is everywhere - sometimes even in yarn! #PiDay #InternationalDayOfMathematics #IDM #MathsArt #WomenInMaths #HerMathsStory

[] Kekkonen, H. (2024). Crocheting Bour’s Minimal Surfaces. Mathematical Intelligencer, 46(4), 306-312. https://doi.org/10.1007/s00283-023-10314-1

A white crocheted Richmond minimal surface on white background

**AdeptVeritatis** @AdeptVeritatis@social.tchncs.de · Mar 9

Mar 9

AdeptVeritatis @AdeptVeritatis@social.tchncs.de

#KaleidoSaturday

#Art #GenerativeArt #kaleidoscope

**AdeptVeritatis** @AdeptVeritatis@social.tchncs.de · Mar 1

Mar 1

AdeptVeritatis @AdeptVeritatis@social.tchncs.de

#KaleidoSaturday

I wanted to release the project files of my animations for a long time. Now you can play around with it, too!

project file:
https://gitlab.freedesktop.org/AdeptVeritatis/visuals/-/blob/main/mix003.json

video mixer app:
https://gitlab.freedesktop.org/AdeptVeritatis/pw-videomix

With a short tutorial in the following posts.

Colorful mandala with fiery red tones and some green in it.

#Art #GenerativeArt #kaleidoscope

**Naomi** @naomih@mathstodon.xyz · Mar 1

Mar 1

Naomi @naomih@mathstodon.xyz

Origami pentakis dodecahedron!

I decided a few weeks ago that I wanted to make a truncated isocahredron out of sonobe units. I made some and started assembling them into triangles and then (what I now know to be) a hexapentakis truncated icosahedron. I soon decided to actually calculate how many sonobe modules I would need and decided 270 was far too many so rethought my plan and settled on a pattern that only requires 90 modules.

I then set various rules for the colours and spent a lot of last week playing with different options and trying to find one which satisfied my conditions. I was working on colouring the graph of a truncated isocahredron and wanted:
(1) all edges around a face to be a different colour to each other and
(2) all edges coming out of a face (spokes?) to be different colours to each other too
(and of course any adjacent edges must be different but this is covered by condition (1)).

After many failed attempts of doing this by hand and beginning to doubt it was possible, I eventually numbered the edges and made this into a graph colouring problem on a graph with 90 vertices and stuck it into Sage which confirmed the chromatic number of the 90-vertex graph was in fact 6 and gave me a possible colouring.

Then several hours later at the end of last week, the modules had been put together. Due to the way each edge from my diagram was rotated, the resulting shape is made of triangular faces (if you imagine filling in the gaps) and is in fact the dual of the truncated icosahedron - i.e. the pentakis dodecahedron.